Project Outline
For my project I would like to build on the work of a previous student, Rahul Khakurel, who also explored Rayleigh scattering. However the form of the Rayleigh theory I will be working with is modified to include different variables. These variables would be easier to measure in an experimental setting and can be manipulated to observe the effect on the scattering intensity.
Link: http://pages.vassar.edu/magnes/applied-quantum-mechanics-and-optics-phys-375/rahul-khakurel/
The Rayleigh theory of light intensity for scattering by a single particle is:
(1) 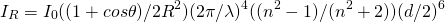
R=distance to the sample, θ=scattering angle, n=refractive index, d=diameter of the particle
The cross section of one particle is:
(2) 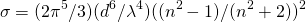
(Zare et al., Laser Experiments for Beginners)
However, I would like to look at the scattering for multiple particles suspended in a solution with water. The equation I will be using is:
(3) 
I0= incident intensity, σ=scattering cross section (same as for single particle), N=particle density, nwater=refractive index of water, l=cuvette length, θ=scattering angle
All of these variables can be manipulated, except for the refractive index of water.
The Rayleigh coefficient, which is the particle cross section multiplied by the particle density of the cuvette., accounts for the likelihood of scattering given particle size and density. The likelihood of scattering is also dependent on the distance the light travels through the sample, or the length of the cuvette. As the light passes through the sample it is also refracted by both the particles and background medium. (For my modeling purposes the background medium is water). The 1+cosine term accounts for the phase shift between the incident light and the scattered light based on the angle between the incident beam and the observed scattered light. The incident intensity is the amount of light entering the cuvette and is also the maximum intensity of light that could be scattered.
I am assuming for the purposes of modeling that the particles do not absorb any of the light and that they are spherical in shape.
Just for reference, here is the equation Rahul was using:
(4) 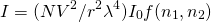
N=number of spheres, V=volume, r=distance to observer, λ=wavelength, I0=incident intensity, f=function taking into account differing refractive indices
For his work he set the distance to the observer, incident intensity, and the function taking into account the differing refractive indices all equal to one.
When the particle size is no longer within the Rayleigh limit the Rayleigh-Debye equation will be used. This equation introduces a unitless form factor to the Rayleigh theory so that the theory can be applied to particles larger than the Rayleigh limit. (Simple alternative to Mie scattering, which accurately predicts scattering for larger particles but is quite complicated.
The Rayleigh-Debye equation:
(5) 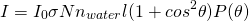
the form factor (Kerker, The Scattering of Light):
(6) ![Rendered by QuickLaTeX.com \begin{equation*} P(\theta)=[(3/u^3)(sin(u)-ucos(u))]^2 \end{equation*}](https://pages.vassar.edu/magnes/wp-content/ql-cache/quicklatex.com-57cc5e68a44f9457a93f9643634985d7_l3.png)
(7) 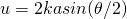
(8) 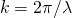
a=particle radius and all the other variables are the same as for the Rayleigh equation
Please Note: In the equation for the form factor the entire function MUST be squared. In Laser Experiments for Beginnings by Richard Zare the form factor equation does not have the squared term. However he references the book by Milton Kerker and the equation is supposed to have a squared. This would explain the weird “tails” of x-axis negative intensity that Rahul’s graphs had. The graphs should have forward x-axis scattering only.
I would like to first make a graph that predicts the how the scattering intensity changes as a function of wavelength for a varying number of particle densities (but a controlled particle size within the Rayleigh limit). This graph will be made using Mathematica and the wavelengths used will be the visible spectrum. The scattering intensity will be measure at 90 degrees—the angle at which there is maximum scattering intensity for Rayleigh scattering.
Previously Rahul modeled the Rayleigh light scattering as the particle size changed from 2nm to 902nm for yellow light. Since Rayleigh scattering is wavelength dependent I would like to show how scattering changes as particle size changes (within the Rayleigh limit) for other wavelengths of light in addition to yellow—like red, green, and blue—to explore whether the change in scattering becomes more for certain wavelengths. The concentration will be held constant. This will also be done using Mathematica.
I will then look at the scattering intensity for the range of particles just under and just over the Rayleigh limit for the same wavelengths used in the graph of particles within the Rayleigh limit using both the Rayleigh and Rayleigh-Debye factor, which introduces a correcting form factor. This is to observe the predicted intensity around the boundary of the Rayleigh limit, and how that intensity varies due to the introduction of the form factor.
The goal of predicting the scattering intensity would be to explore Static Light Scattering. Static Light Scattering uses the scattering intensity predicted by the Rayleigh theory (at a 90 degree scattering angle) and the concentration of the cuvette to determine the molecular weight of the particles. Molecular weight can be measured, in theory, by creating a Debye plot (Intensity of scattered light vs. concentration) and finding the intercept at zero concentration (which is equal to 1/molecular weight). The gradient of the Debye plot is the 2nd Viral Coefficient (A2), which can be used to determine the interaction strength between the solvent and the particles.
A2 >0 the particles will stay in stable solution
A2 < 0 the particles may aggregate
A2 = 0 the particle-solvent interactions are completely balanced by the particle-particle interactions
Applications of Static Light Scattering:
Static Light Scattering has experimental application because it could be used to determine the weight of the molecules in an unknown solution. By comparing the experimentally determined weight to predicted molecular weights, it may be possible to determine the identity of the molecules. And particle aggregation can be applied to proteins. Protein aggregation can be used as an important marker in many diseases. A “normal” solution taken from a healthy individual can be used as a standard and compared to solutions taken from patients as an early detection method (if the protein aggregates indicates disease).
There are a couple quick checks to determine if the Rayleigh theory is accurate. The first would be to look at the predicted scattering intensity. This intensity should not be greater than the incident intensity. Another check would be that the predicted scattering intensity for the Rayleigh theory is greatest at 90 degrees. For the Rayleigh-Debye equation the forward scattering should be the greatest. Both the Rayleigh theory and Rayleigh-Debye theory can be checked experimentally by actually making a solution and measuring the scattering intensity for a given wavelength. The experimental parameters would provide values for the variables in the Rayleigh (and Rayleigh-Debye) equation. With those values the predicted scattering intensity could be determined and compared to the actual scattering intensity. In order to check the Static Light Scattering, the molecular weight of a particle with a known molecular weight could be predicted and then compared to that known weight.
REFERENCES:
Kerker, Milton. The Scattering of Light and Other Electromagnetic Radiation. New York: Academic Press, 1969
Zare, Richard N., Bertrand H. Spencer, Dwight S. Springer, Matthew P. Jacobson. Laser Experiments for Beginners. Sausalito: University Science Books, 1995.
![]()
![]()
![]()
![]()
![]()