Measuring and Modeling Physical RCL Circuits

Extended Derivations of LC and RLC circuit behavior.

To derive the equations governing the voltage across the capacitor I start with Kirchoff’s Loop Law:

$\Delta V_{L}+\Delta V_{C}=0$ (1)

Substituting in for the values of voltage across a capacitor and inductor we get:

$L\dfrac {dI\left( t\right) } {dt}+\dfrac {Q\left( t\right) } {C} =0$ (2)

The current can be expressed as the rate of change of charge on the capacitor:

$I\left( t\right) =\dfrac {dQ\left( t\right) } {dt}$ (3)

$L\dfrac {d^{2}Q\left( t\right) } {dt^{2}}+\dfrac {Q\left( t\right) } {c}=0$ (4)

This expression is analogous to the equations governing Simple Harmonic Motion (SHM):

$F_{spring}=-kx$ (5)

$F_{net}=ma= m\dfrac {d^{2}x\left( t\right) } {dt^{2}}$ (6)

$m\dfrac {d^{2}x\left( t\right) } {dt^{2}}+kx =0$ (7)

The solution to this second order differential equation can be written as.

$x\left( t\right) =x_{0}\sin \left( \omega _{0}t-\delta \right)$ (8)

Similarly the general solution for charge across the capacitor can be given by:

$Q\left( t\right) =Q_{0}\sin \left( \omega _{0}t-\delta \right)$ (9)

Thus the Voltage across the capacitor, $\Delta V=\dfrac {Q\left( t\right) } {C}$ , can be written as:

$\Delta V\left( t\right)=\dfrac {Q_{0}} {C}sin \left( \omega _{0}t-\delta \right)\left$ (10)

$\Delta V\left( t\right)=V_{0}sin \left( \omega _{0}t-\delta \right)\left$ (11)

RLC circuit (damped oscillation)

The RCL circuit can be similarly derived by adding a third term for the voltage across the resistor to Kirchoff’s Loop Law.

$\Delta V_{L}+\Delta V_{R}+\Delta V_{C}=0$ (12)

$L\dfrac {d^{2}Q\left( t\right) } {dt^{2}}+R\dfrac {dQ\left( t\right) } {dt}+\dfrac {Q\left( t\right) } {c}=0$ (13)

$\dfrac {d^{2}Q\left( t\right) } {dt^{2}}+\dfrac {R} {L}\dfrac {dQ\left( t\right) } {dt}+\dfrac {1} {LC}Q\left( t\right) =0$ (14)

Here we define

$\dfrac {1} {LC}\equiv \omega _{0}^{2}$ and $\dfrac {R} {L}\equiv 2\beta$ (15)

To simplify our second order differential equation to:

$\dfrac {d^{2}Q\left( t\right) } {dt^{2}}+2\beta \dfrac {dQ\left( t\right) } {dt}+\omega _{0}^{2}Q\left( t\right) =0$ (16)

Again, this equation is analogous to Damped Simple Harmonic Motion, described below:

$F_{spring}=-kx -b\dfrac {dx\left( t\right) } {dt}$ (17)

$F_{net}=ma= m\dfrac {d^{2}x\left( t\right) } {dt^{2}}$ (18)

$m\dfrac {d^{2}x\left( t\right) } {dt^{2}}+kx -b\dfrac {dx\left( t\right) } {dt} =0$ (19)

The general solution to this differential equation, describing an underdamped system (where $\beta < \omega _{0}$ ), is as follows:

$\omega _{1}\equiv \sqrt {\omega _{0}^{2}-\beta ^{2}}$ (20)

$V\left( t\right) =V_{0}e^{-\beta t}\sin \left( \omega _{1}t-\delta \right)$ (21)

This solution describes a sinusoidal function whose amplitude decays as a function of $e^{-\beta t}$ .

While in theory my experimental setup is an LC circuit, and should be described by SHM, in reality there should be some form of resistance in the circuit, causing the oscillations to decay over time as described in Damped SHM.

Experimental Setup:

EM-schematics

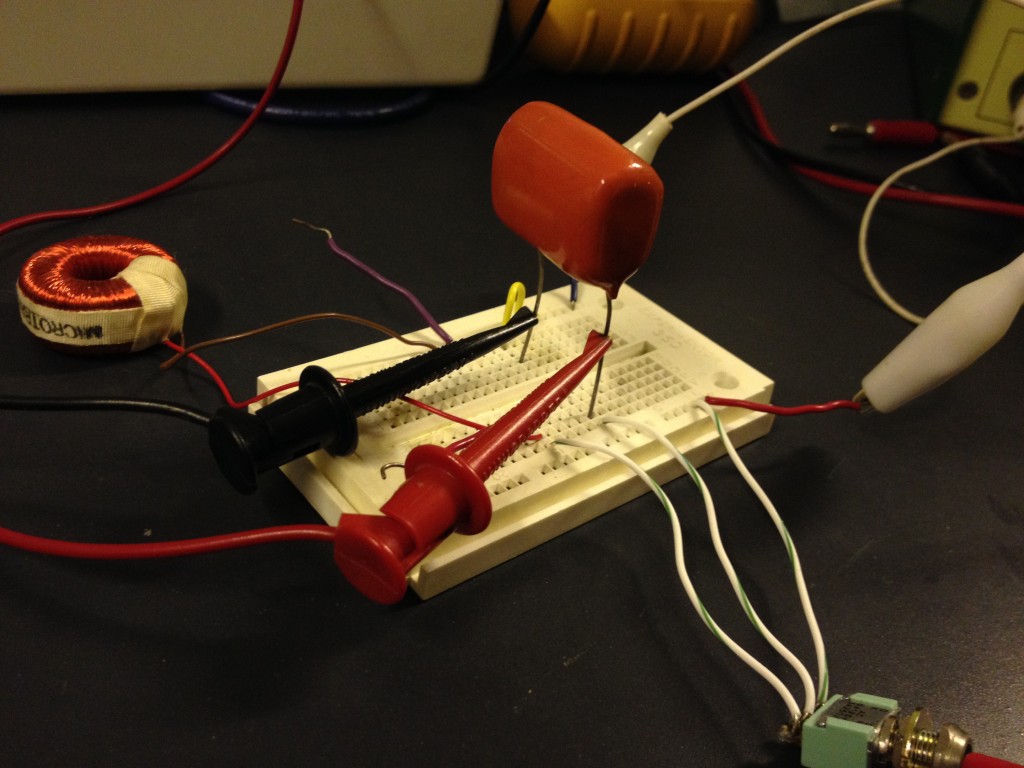
The above diagram represent the physical circuit I built to observe the voltage oscillations of an LC circuit. This circuit has two functions. The first, when the switch connects the loop to the left, charges the capacitor with the voltage source. The second, when the switch completes the right loop, discharges the capacitor through the inductor. The right and left loops are independent of each other because of the switch. Connected in parallel across the capacitor is the PicoScope. This device records the voltage across the capacitor over time. Below are photographs of what experimental setup actually looked like:

DC power source, signal generator, oscilloscope, circuit (and multimeter).

Circuit setup. LC circuit with DC power source, switch, capacitor, inductor, and oscilloscope.

Oscilloscope with Channel A: Voltage difference, Channel B: reference signal.

First Data:

This project held a few challenges of its own before I even got to collecting data. Completely unfamiliar with the Picoscope (digital oscilloscope) probe and software, it took me a few days to figure out how to efficiently collect data with the setup.

My first attempt at taking data did not go well. With the oscilloscope I could see the voltage difference across the capacitor that I wanted, but when I switched the circuit to discharge the capacitor, the voltage dropped to zero immediately. I was looking for some sort of sinusoidal curve, possibly decaying very quickly (in ideal conditions the curve would never decay).

Searching for answers as to why I wasn’t seeing anything, attempted to estimate how long any capacitor would take to discharge.

The charge on a capacitor as a function of time (in an RC circuit) is given by $Q=Q_{0}e^{-tk}$ , where $k=\dfrac {1} {RC}$ . At the time I was using components of unknown value. I visited Larry Doe’s workshop in Blodget and measured the resistance, capacitance, and inductance values of all my components. The capacitor I had picked for my initial measurements had a value of 0.1 μF. What this means is the charge on the capacitor had discharged $e^{-1}$ of it’s initial value (about 37% of it’s initial value) at $t=RC$ . In this scenario the R is the resistance of the circuit. Using an estimation of 0.0001 Ω for 5 cm copper wire as the equivalent resistance of the circuit, and 10^-7 F as the capacitance, the charge on the capacitor should reach one third it’s initial value in 10 ps. To me this illustrated the incredibly short time period at which that particular LC combination would oscillate, and potentially decay.

Second Attempt:

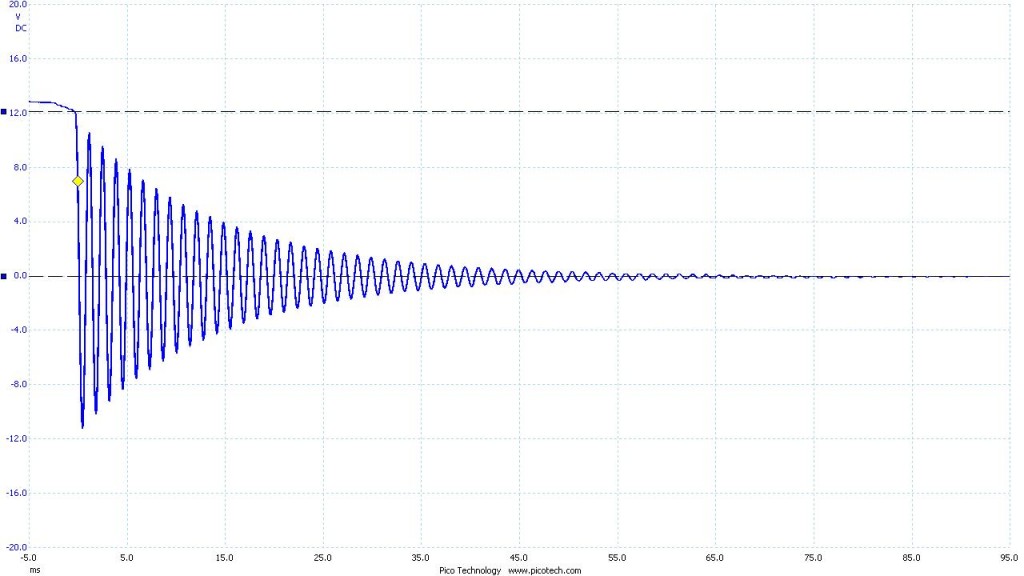
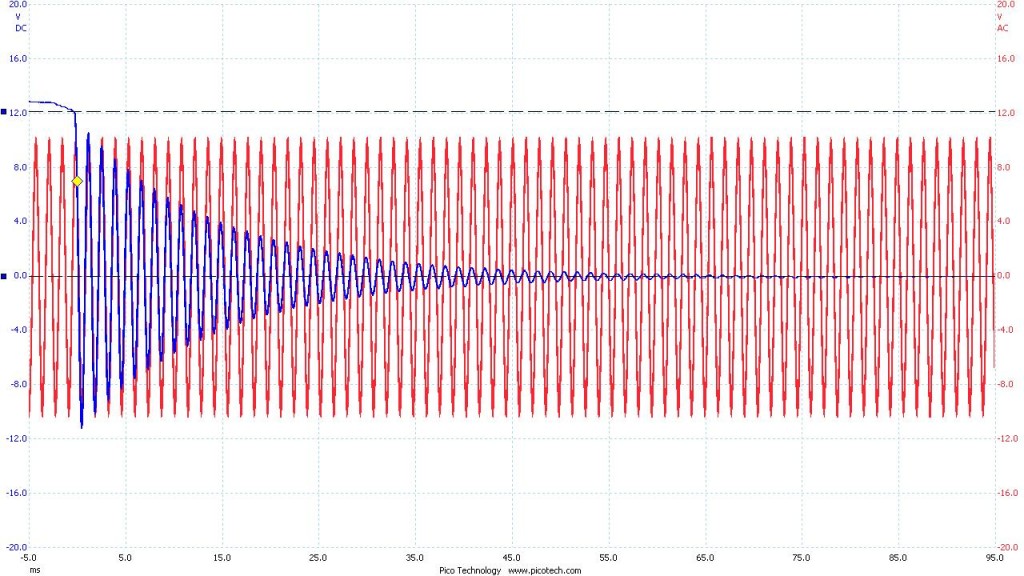
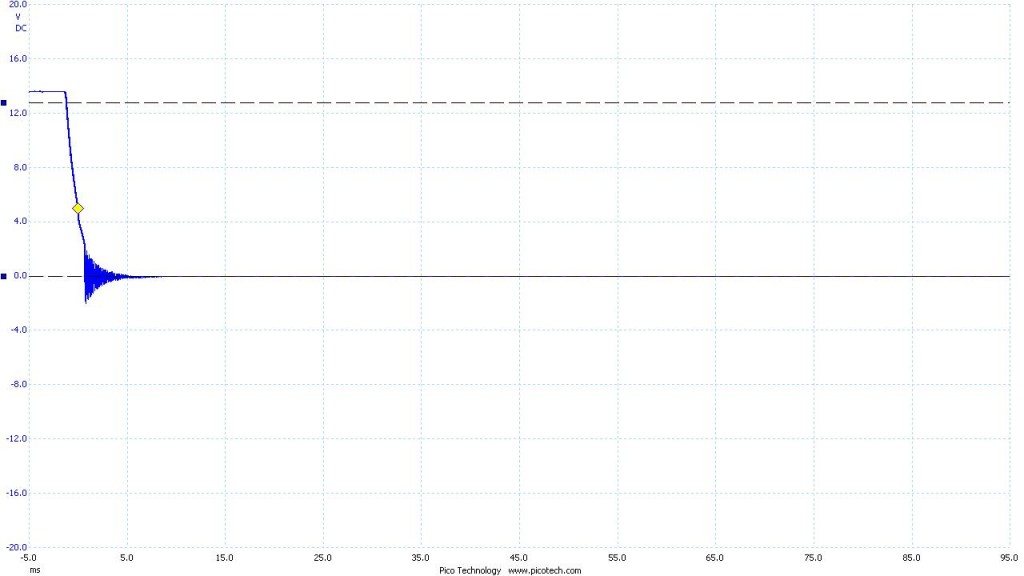
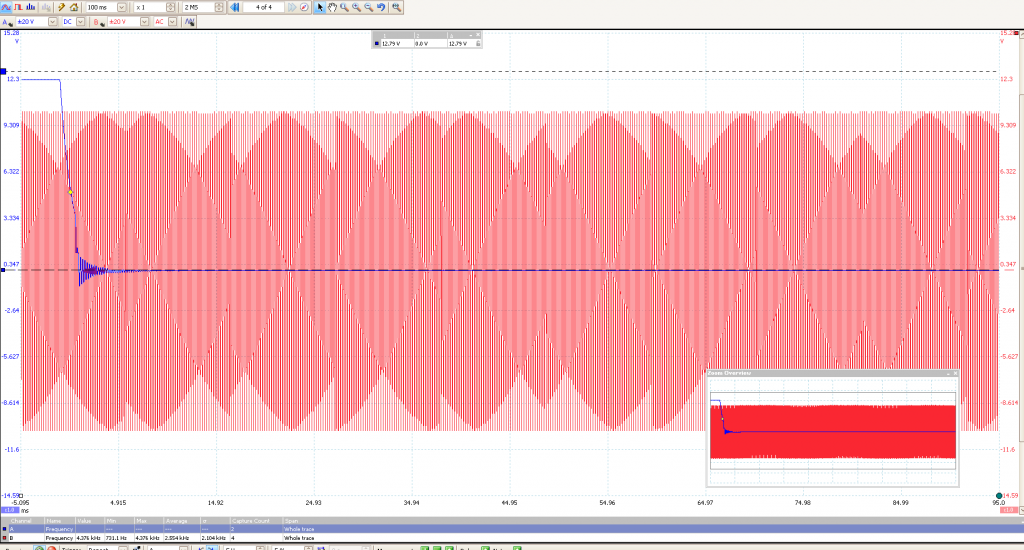
To maximize the time it took the system to discharge I used the highest valued capacitor and inductor I had. The resulting curve was far more manageable. Below is the data I collected with the oscilloscope. The blue curve you see is voltage across the capacitor as a function of time. The total time shown for each image is 100 ms. The red curve is an artificially generated curve for reference. It represents the expected shape of the blue curve. In an ideal LC circuit there is no resistor, and thus no decay over time. Theoretically an ideal LC circuit would oscillate indefinitely.

Figure 1. Capacitor: 1465 nF Inductor: 996 mH

1465nF, 996mH, Oscillation: 128 Hz

Figure 1.1 With reference wave

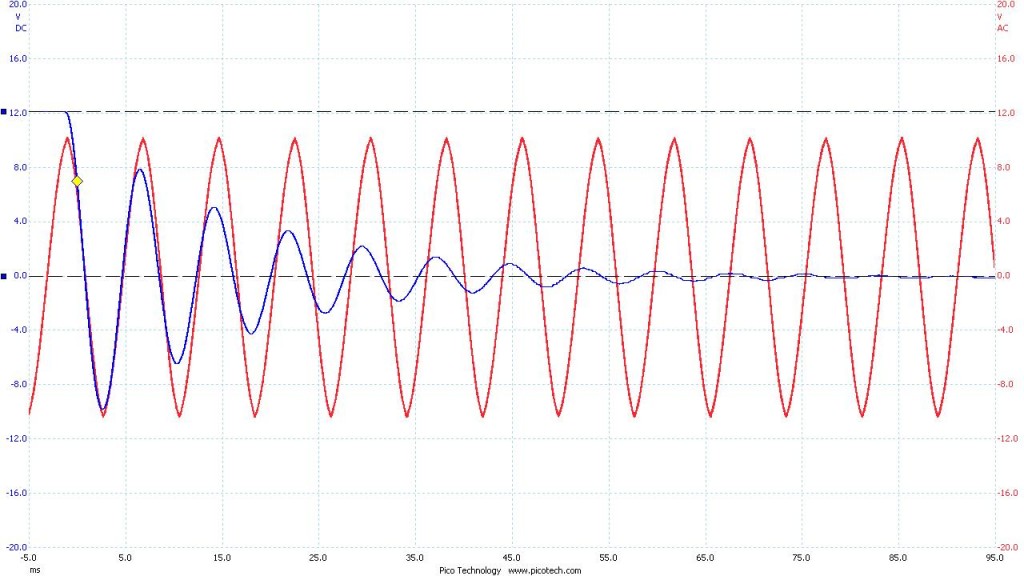
Oscillation: 128 Hz

I then used successively lower and lower capacitor values to approach my initial test where the capacitor discharge was far too quick to measure. The duration of measurement, initial voltage across the capacitor, and inductor value were held constant.

Figure 2. Capacitor: 1007 nF Inductor: 996 mH

1007nF, 996mH, Oscillation: 155 Hz

Figure 2.1 With reference wave

Oscillation: 155 Hz

Figure 3. Capacitor: 47 nF Inductor: 996 mH

47nF, 996mH, Oscillation: 730 hZ

Figure 3.1 With reference wave

Oscillation: 730 hZ

Figure 4. Capacitor: 1.9 nF Inductor: 996 mH

1.9nF, 996mH, Oscillation: 4.23 kHz

Figure 4.1 With reference wave

Oscillation: 4.23 kHz

2 thoughts on “Preliminary Data”

John August 6, 2014 at 12:26 am

Stumbled upon your blog. Very interesting derivation. I specially like the part that you used the Picoscope to verify it. Thank you for sharing.
Jenny Magnes May 19, 2014 at 4:28 pm

This is good evolution of data collection. Definitely label your x-axis. How would your graphs change if you just changed the capacitor or just the inductor values. Could you produce a frequency versus inductance or capacitance graph?

Comments are closed.

Modeling and Experimental Tools with Prof. Magnes

Ideal for getting ideas and feedback on upper level physics tools.

Preliminary Data