Second Harmonic Generation is a special case of optical mixing. It is a process by which photons from a laser beam are mixed in a nonlinear medium and the output photon has double the energy and frequency and half the wavelength. Conditions satisfy $\omega_{1}=\omega_{2}=\omega$ and $\omega_{3}=2\omega$. Both energy and momentum conservation must be satisfied. Energy by $\omega_{3}=\omega_{1}=\omega_{2}$ and momentum by $k_{3}=k_{1}+ k_{2}$
In more detail:
“Nonlinear optics is the study of phenomena that occur as a consequence of the modification of the optical properties of a material system by the presence of light”. Input waves are at frequencies $\omega_{1}$ and $\omega_{2}$. By the nonlinear effects of incident beams (at the atomic level) each atom develops an oscillating dipole moment which contains a component at frequency $\omega_{1}+\omega_{2}$. Each atom radiates this frequency but there are many atoms in our medium and hence many atomic dipoles oscillating with a phase determined by the phases of the incident waves. When the relative phasing matches the waves radiated by each dipole will add constructively turning the system into a phased array of dipoles. When this happens the electric field strength of the radiation emitted will be the number of atoms times larger and hence the intensity will be the number of atoms squared.
I will assume my system to be lossless and dispersion-less for simplifying equations
(1) ![]()
2.1.19 Nonlinear optics Robert W.Boyd
$\widetilde{P}^{NL}_{n} =$ Non-Linear part of Polarization Vector
$ \widetilde{E}_{n} =$ Electric Field vector
$\epsilon^{(1)}(\omega_{n}) =$ Frequency dependent dielectric Tensor
Equation (1) is derived from Maxwell’s equation and is the equation for waves in medium. It is valid for each frequency component of the field.
$\widetilde{E}_{2}(z,t) =A_{2}e^{i(k_{2}z-wt)}, \widetilde{P}_{j}(z,t) =P_{j}e^{-i\omega_{j}t},$ $P_{1} =4dA_{2}A^{*}_{1}e^{i(k_{2}-k_{1})z}, P_{2} =2dA^{2}_{1}e^{i2k_{1}z}$
2.2.1, 2.2.3, 2.2.4,2.2.5, 2.2.7 Nonlinear optics Robert W.Boyd
$\widetilde{E}_{2}(z,t)$ will be my equation for the transmitted wave at frequency propagating in the z direction,$ \widetilde{P}_{2}(z,t)$ the nonlinear source term and $P_{2}, P_{1}$ the amplitude of the nonlinear polarization and amplitude of incident beam respectively. I will make diagrams of incident waves hitting the medium and resulting transmitted wave.
Substituting the transmitted wave equation in the wave equation and solving by hand I will find coupled amplitude equation.
(2) ![]()
2.6.11 Nonlinear optics Robert W.Boyd
$\bigtriangleup k = k_{1}+k_{1}-k_{2}$ is the wave vector mismatch
2.6.12 Nonlinear optics Robert W.Boyd
$A_{i} =$ amplitude of the wave
The coupled amplitude equation shows how the amplitude of $\omega_{2}$ wave varies due to it’s coupling of two $\omega_{1}$ waves. And from this we can find intensity, which is more useful.
![]()
(3) 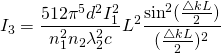
2.2.17, 2.20 Nonlinear optics Robert W.Boyd
Where $\lambda_{2}=\frac{2\pi c}{\omega_{2}}$ , L is the length of the medium and d is the tensor
I will try and model the effect of $\bigtriangleup k$ of the wave vector on the efficiency and take special notice when $\bigtriangleup k=0$ since this is the condition for perfect phase matching. I will make an animation to vary $(\frac{\bigtriangleup kL}{2})$ in the intensity equation and this will show the effects of wave vector mismatch on the efficiency of harmonic-generation.
I will also attempt to model the effects of absorption.
As an example I will use the nonlinear media KDP (Potassium Dihydrogen Phospate) crystal to model second harmonic generation for a laser beam at $1.06\mu$ meters. KDP is widely used in commercial Non linear optical materials because of its electro-optic effects and it’s high non-linear coefficients.
REFERENCES:
Boyd. Nonlinear Optics. New York: Academic Press, 1992
SHEN. The Principles of Nonlinear Optics. New York: Wiley-Interscience, 1984


Your project sounds very interesting, but also very confusing at the same time. First of all, could you explain what a nonlinear medium is? It would be nice to have an idea of what kind of material all of this is taking place within. Also, I’m confused about how you said that energy must be conserved, but if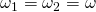 and
and 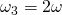 , then how can
, then how can 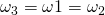 ? It seems like this whole project is based upon finding the relationship between how the waves interfere with one another and its relation to the difference in wave vector,
? It seems like this whole project is based upon finding the relationship between how the waves interfere with one another and its relation to the difference in wave vector, 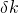 . This seems to be your focus, but perhaps if you explain your equations more, the average person will be able to understand the methods you follow throughout your project!
. This seems to be your focus, but perhaps if you explain your equations more, the average person will be able to understand the methods you follow throughout your project!
Interesting and good referencing. Be sure to explain ALL variables. How is it that delta k relates to the efficiency? What is KDP? Will you solve this differential equation by hand? How will the coupled equations reveal delta k?